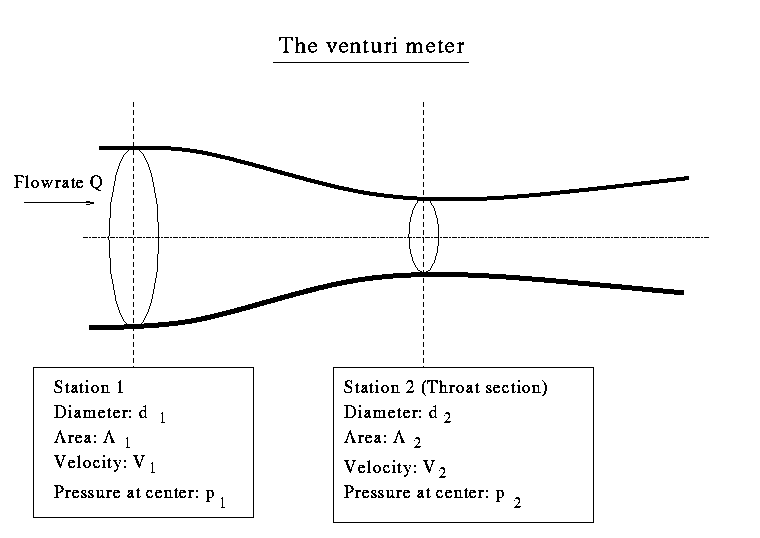
Application of Bernoulli equation to venturi meter (device used to measure flowrate) (© Kinnas 2000)
Applying the Bernoulli equation between the shown stations (1) and (2) along the center we get:
Assuming that the venturi meter is horizontal ( 
), and that the flow
is uniform at both stations (thus: 
and 
), the above
equation becomes:
Solving the above equation with respect to Q we get:
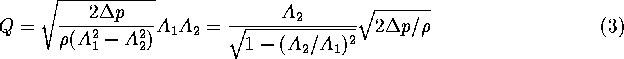
The above equation relates the flowrate Q to the pressure
differential 
. This pressure differential can be measured
(as in the case of the Pitot tube we described in class)
through pressure taps (holes) on the walls of the venturi meter,
at stations (1) and (2) that are connected with the sides of a U-manometer,
as shown on p. 451 of the textbook. At the same section on p. 451
in the textbook a modified formula for Q is given which also includes the effects
of viscous forces (which are completely ignored when applying the
Bernoulli equation). The venturi meter is covered in more detail
in advanced Fluid Mechanics courses.
A similar venturi is used in the applet on the web. We have created this applet to help you understand the definitions of the piezometric and the total head.